[LeetCode 123] Best Time to Buy and Sell Stock III

Question Link:

https://leetcode.com/problems/best-time-to-buy-and-sell-stock-iii/

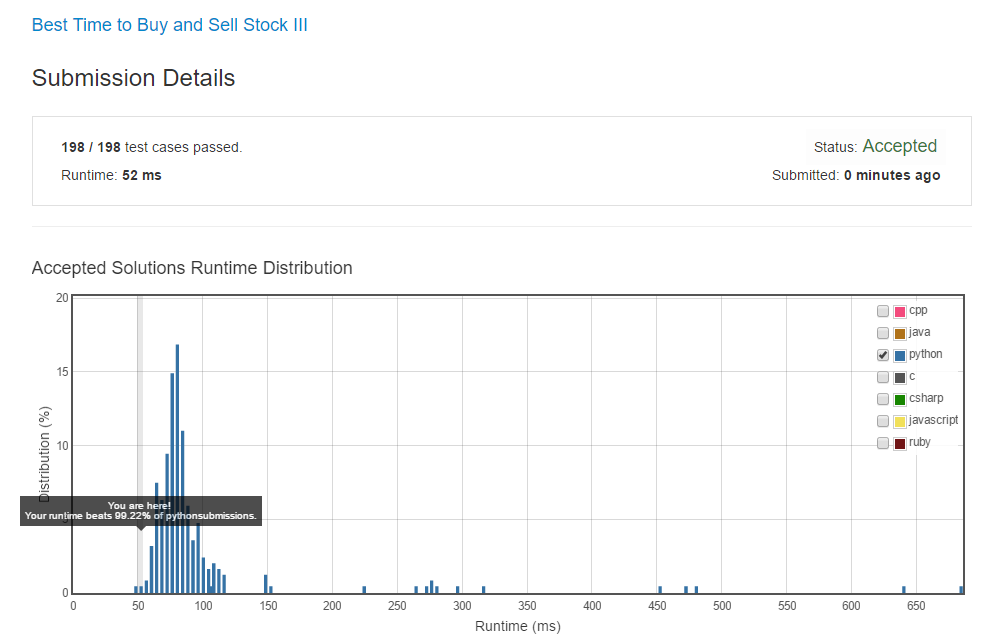
人生新高，99.22%，亲啊，这是真的么……

## Linear Time Solution

We try to solve this problem in O(n) time by using the solution of Best Time to Buy and Sell Stock, which finds the optimal profit of one transaction.

Suppose the transaction (b, s) is the optimal for price list P[1..n]. Then we have:

1. P[i] >= P[b], for i = 1, ..., s
2. P[i] <= P[s], for i = b, ..., n

Let find_best_transaction is the function to find optimal transaction, the following algorithm solves the problem:

1. By given the price list P[1..n], find the best transaction (b,s) = find_best_transaction(P)
2. Find the maximum profit for the following three parts divided by b and s:
   • P1: the maximum profit for the price list P[1..b-1]
   • P2: the maximum profit for the price list P[b+1..s-1].inverse()
   • P3: the maximum profit for the price list P[s+1..n]
3. The answer should be P[s] - P[b] + max(P1,P2,P3).

Correctness

Now we prove the algorithm above is correct. Let (b,s) be the optimal transaction of P[1..n], then there are two cases: 1) (b,s) is one of the two transactions; or 2) (b,s) is not in the result.

For case 1), according to the problem description, you must sell the stock before you buy again, so the other transaction must happen in P[1...b+1] or P[s+1..n]. Then the answer is (P[s] - P[b]) + max(P1, P3).

For case 2), let (b1, s1) and (b2, s2) be the first and second transactions of the solution, where 1 <= b1 < s1 < b2 < s2 <= n. We can have following cases:

Case 2-1: s < b2

The optimal answer could be (b, s) and (b2, s2), because we can replace (b1, s1) with (b, s). Therefore the answer of this case could be P[s] - P[b] + P1

Case 2-2: b > s1

The optimal answer could be (b1, s1) and (b, s), because we can replace (b2, s2) with (b, s). Therefore the answer of this case could be P[s] - P[b] + P2

Case 2-3: b <= s1 and s >= b2

This means b <= s1 < b2 <= s, then we have:

1. We can replace b1 with b because P[b] is the minimum of P[1..s];
2. We can replace s2 with s because P[s] is the maximum of P[b..n].

The optimal solution could be like: --- b --- s1 --- b2 -- s --- for this case, where the profit is P[s1] - P[b] + P[s] - P[b2], that is, P[s] - P[b] + P[s1] - P[b2]. And P[s1] - P[b2] is just the answer of find the optimal transaction of P[s-1 .. b+1]. Therefore the answer of this case could be P[s] - P[b] + P2

Considering all cases above, the optimal profit should be maximum of all four cases, which is P[s] - P[b] + max(P1, P2, P3).

Python Implement

class Solution(object):
    def maxProfit(self, prices):
        """
        :type prices: List[int]
        :rtype: int
        """
        n = len(prices)
        if n < 2:
            return 0

        # Find the best transaction
        b, s, profit = self.find_best_transaction(prices)

        # Find max(P1, P2, P3)
        profit1 = 0
        if b > 1:
            profit1 = max(profit1, self.find_best_transaction(prices[:b])[2])
        if s > b + 2:
            profit1 = max(profit1, self.find_best_transaction(prices[b+1:s][::-1])[2])
        if s < n - 2:
            profit1 = max(profit1, self.find_best_transaction(prices[s+1:])[2])

        # Return
        return profit + profit1

    def find_best_transaction(self, prices):
        """
        Return the optimal transaction (b, s) of prices.
        """
        b = s = l = 0
        for i in xrange(1, len(prices)):
            if prices[i] <= prices[l]:
                l = i
            elif prices[i] - prices[l] > prices[s] - prices[b]:
                b = l
                s = i
        return b, s, prices[s] - prices[b]